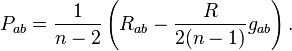Bach tensor

In differential geometry and general relativity, the Bach tensor is a trace-free tensor of rank 2 which is conformally invariant in dimension n = 4.^[1] Before 1968, it was the only known conformally invariant tensor that is algebraically independent of the Weyl tensor.^[2] In abstract indices the Bach tensor is given by

B_{ab} = P_{cd}{{{W_a}^c}_b}^d+\nabla^c\nabla_aP_{bc}-\nabla^c\nabla_cP_{ab}

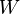where $W$ is the Weyl tensor, and 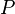$P$ the Schouten tensor given in terms of the Ricci tensor 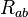$R_{ab}$ and scalar curvature 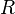$R$ by

P_{ab}=\frac{1}{n-2}\left(R_{ab}-\frac{R}{2(n-1)}g_{ab}\right).

See also

References

↑ Rudolf Bach, "Zur Weylschen Relativitätstheorie und der Weylschen Erweiterung des Krümmungstensorbegriffs", Mathematische Zeitschrift, 9 (1921) pp. 110.
↑ P. Szekeres, Conformal Tensors. Proceedings of the Royal Society of London. Series A, Mathematical and Physical Sciences Vol. 304, No. 1476 (Apr. 2, 1968), pp. 113–122

Further reading

Arthur L. Besse, Einstein Manifolds. Springer-Verlag, 2007. See Ch.4, §H "Quadratic Functionals".
Demetrios Christodoulou, Mathematical Problems of General Relativity I. European Mathematical Society, 2008. Ch.4 §2 "Sketch of the proof of the global stability of Minkowski spacetime".
Yvonne Choquet-Bruhat, General Relativity and the Einstein Equations. Oxford University Press, 2011. See Ch.XV §5 "Christodoulou-Klainerman theorem" which notes the Bach tensor is the "dual of the Coton tensor which vanishes for conformally flat metrics".
Thomas W. Baumgarte, Stuart L. Shapiro, Numerical Relativity: Solving Einstein's Equations on the Computer. Cambridge University Press, 2010. See Ch.3.

This article is issued from Wikipedia - version of the 12/2/2013. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.