Conway criterion
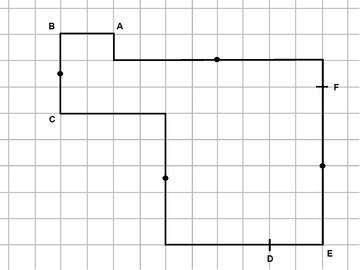
In the mathematical theory of tessellations, the Conway criterion, named for the English mathematician John Horton Conway, describes rules for when a prototile will tile the plane; it consists of the following requirements:[1] The tile must be a closed topological disk with six consecutive points A, B, C, D, E, and F on the boundary such that:
- the boundary part from A to B is congruent by translation to the boundary part from E to D
- each of the boundary parts BC, CD, EF, and FA is centrosymmetric—that is, each one is congruent to itself when rotated by 180-degrees around its midpoint
- some of the six points may coincide but at least three of them must be distinct.[2]
Any prototile satisfying Conway's criterion admits a periodic tiling of the plane—and does so using only translation and 180-degree rotations. The Conway criterion is a sufficient condition to prove that a prototile tiles the plane but not a necessary one; there are tiles that fail the criterion and still tile the plane.[3]
Examples


In its simplest form the criterion states that any hexagon with a pair of opposite sides that are parallel and congruent will tessellate the plane by translation, called hexagonal parallelogons.[4] But when some of the points coincide, the criterion can apply to other polygons and even to shapes with curved perimeters.[5]
The Conway criterion discriminates many shapes, especially polyforms: except the two tiling nonominoes on the right, all tiling polyominos up to the nonominoes can form a patch of at least one tile which satisfies the criterion.[3] These figures also show that the criterion is a sufficient but not necessary condition for a prototile to tile the plane.
References
- ↑ Will It Tile? Try the Conway Criterion! by Doris Schattschneider Mathematics Magazine Vol. 53, No. 4 (Sep., 1980), pp. 224-233
- ↑ Periodic Tiling: Polygons in General
- 1 2 Planar tilings by polyominoes, polyhexes, and polyiamonds by Glenn C. Rhoads, Journal of Computational and Applied Mathematics Vol 174, Issue 2, 15 (Feb 15, 2005), pp. 329–353
- ↑ Polyominoes: A Guide to Puzzles and Problems in Tiling, by George Martin, Mathematical Association of America, Washington, DC, 1991, p. 152, ISBN 0883855011
- ↑ The five types of Conway Criterion polygon tile, PDF file
External links
- History and introduction to polygon models, polyominoes and polyhedra, by Anthony J Guttmann
- G C Rhoads (2005) Planar tilings by polyominoes, polyhexes, and polyiamonds, Journal of Computational and Applied Mathematics, V 174 p 329-353
| ||||||||||||||||||||||||||||||||||||||
