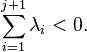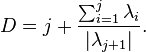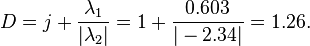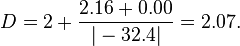Kaplan–Yorke conjecture

In applied mathematics, the Kaplan–Yorke conjecture concerns the dimension of an attractor, using Lyapunov exponents.^[1]^[2] By arranging the Lyapunov exponents in order from largest to smallest 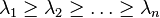$\lambda_1\geq\lambda_2\geq\dots\geq\lambda_n$ , let j be the index for which

\sum_{i=1}^j \lambda_i > 0

and

\sum_{i=1}^{j+1} \lambda_i < 0.

Then the conjecture is that the dimension of the attractor is

D=j+\frac{\sum_{i=1}^j\lambda_i}{|\lambda_{j+1}|}.

Examples

Especially for chaotic systems, the Kaplan–Yorke conjecture is a useful tool in order to determine the fractal dimension of the corresponding attractor.^[3]

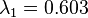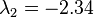The Hénon map with parameters a = 1.4 and b = 0.3 has the ordered Lyapunov exponents $\lambda_1=0.603$ and $\lambda_2=-2.34$ . In this case, we find j = 1 and the dimension formula reduces to

D=j+\frac{\lambda_1}{|\lambda_2|}=1+\frac{0.603}{|-2.34|}=1.26.

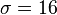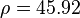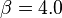The Lorenz system shows chaotic behavior at the parameter values $\sigma=16$ , $\rho=45.92$ and $\beta=4.0$ . The resulting Lyapunov exponents are {2.16, 0.00, -32.4}. Noting that j = 2, we find

D=2+\frac{2.16 + 0.00}{|-32.4|}=2.07.

References

↑ J. Kaplan and J. Yorke, "Chaotic behavior of multidimensional difference equations," in: Functional Differential Equations and the Approximation of Fixed Points, Lecture Notes in Mathematics, vol. 730, H.O. Peitgen and H.O. Walther, eds. (Springer, Berlin), p. 228.
↑ P. Frederickson, J. Kaplan, E. Yorke and J. Yorke, "The Lyapunov Dimension of Strange Attractors," J. Diff. Eqs. 49 (1983) 185.
↑ A. Wolf, A. Swift, B. Jack, H. L. Swinney and J.A. Vastano "Determining Lyapunov Exponents from a Time Series," Physica 16D, 1985, 16, pp. 285–317.

This article is issued from Wikipedia - version of the 3/9/2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.