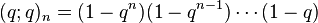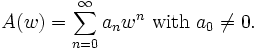q-difference polynomial
In combinatorial mathematics, the q-difference polynomials or q-harmonic polynomials are a polynomial sequence defined in terms of the q-derivative. They are a generalized type of Brenke polynomial, and generalize the Appell polynomials. See also Sheffer sequence.
Definition
The q-difference polynomials satisfy the relation
where the derivative symbol on the left is the q-derivative. In the limit of 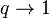 , this becomes the definition of the Appell polynomials:
, this becomes the definition of the Appell polynomials:
Generating function
The generalized generating function for these polynomials is of the type of generating function for Brenke polynomials, namely
where 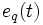 is the q-exponential:
is the q-exponential:
Here, ![[n]_q!](../I/m/4068da6f34e0edc79705024cdc294e3d.png) is the q-factorial and
is the q-factorial and
is the q-Pochhammer symbol. The function 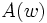 is arbitrary but assumed to have an expansion
is arbitrary but assumed to have an expansion
Any such 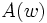 gives a sequence of q-difference polynomials.
gives a sequence of q-difference polynomials.
References
- A. Sharma and A. M. Chak, "The basic analogue of a class of polynomials", Riv. Mat. Univ. Parma, 5 (1954) 325-337.
- Ralph P. Boas, Jr. and R. Creighton Buck, Polynomial Expansions of Analytic Functions (Second Printing Corrected), (1964) Academic Press Inc., Publishers New York, Springer-Verlag, Berlin. Library of Congress Card Number 63-23263. (Provides a very brief discussion of convergence.)
![\left(\frac {d}{dz}\right)_q p_n(z) =
\frac{p_n(qz)-p_n(z)} {qz-z} = \frac{q^n-1} {q-1} p_{n-1}(z)=[n]_qp_{n-1}(z)](../I/m/f936e7309fed49eefb0ba9fac5bbecd8.png)
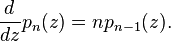
![A(w)e_q(zw) = \sum_{n=0}^\infty \frac{p_n(z)}{[n]_q!} w^n](../I/m/80c5fd994598a3b543cc1d6064b53598.png)
![e_q(t)=\sum_{n=0}^\infty \frac{t^n}{[n]_q!}=
\sum_{n=0}^\infty \frac{t^n (1-q)^n}{(q;q)_n}.](../I/m/9d979f89b4a91c2c63974ee2cda61551.png)