Step function

This article is about a piecewise constant function. For the unit step function, see Heaviside step function.

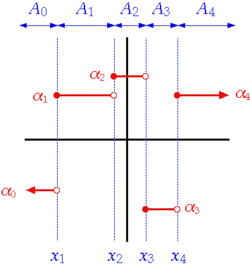
In mathematics, a function on the real numbers is called a step function (or staircase function) if it can be written as a finite linear combination of indicator functions of intervals. Informally speaking, a step function is a piecewise constant function having only finitely many pieces.

Example of a step function (the red graph). This particular step function is right-continuous.

Definition and first consequences

A function $f:\mathbb {R} \rightarrow \mathbb {R}$ is called a step function if it can be written as

f(x)=\sum \limits _{i=0}^{n}\alpha _{i}\chi _{A_{i}}(x)\,

for all real numbers

x

where $n\geq 0,$ $\alpha _{i}$ are real numbers, $A_{i}$ are intervals, and $\chi _{A}\,$ (sometimes written as $1_{A}$ ) is the indicator function of $A$ :

\chi _{A}(x)={\begin{cases}1&{\mbox{if }}x\in A,\\0&{\mbox{if }}x\notin A.\\\end{cases}}

In this definition, the intervals $A_{i}$ can be assumed to have the following two properties:

The intervals are pairwise disjoint, $\scriptstyle A_{i}\,\cap \,A_{j}~=~\emptyset$ for $\scriptstyle i~\neq ~j$
The union of the intervals is the entire real line, $\scriptstyle \cup _{i=0}^{n}A_{i}~=~\mathbb {R} .$

Indeed, if that is not the case to start with, a different set of intervals can be picked for which these assumptions hold. For example, the step function

f=4\chi _{[-5,1)}+3\chi _{(0,6)}\,

can be written as

f=0\chi _{(-\infty ,-5)}+4\chi _{[-5,0]}+7\chi _{(0,1)}+3\chi _{[1,6)}+0\chi _{[6,\infty )}.\,

Examples

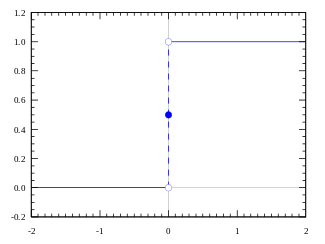
The Heaviside step function is an often-used step function.

A constant function is a trivial example of a step function. Then there is only one interval, $A_{0}=\mathbb {R} .$
The sign function $\operatorname {sgn}(x)$ , which is −1 for negative numbers and +1 for positive numbers, and is the simplest non-constant step function.
The Heaviside function H(x), which is 0 for negative numbers and 1 for positive numbers, is an important step function, and is equivalent to the sign function, up to a shift and scale of range ( $H=(\operatorname {sgn} +1)/2$ ). It is the mathematical concept behind some test signals, such as those used to determine the step response of a dynamical system.

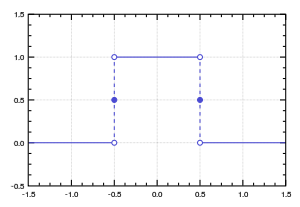
The rectangular function, the next simplest step function.

The rectangular function, the normalized boxcar function, is the next simplest step function, and is used to model a unit pulse.

Non-examples

The integer part function is not a step function according to the definition of this article, since it has an infinite number of intervals. However, some authors^[1] also define step functions with an infinite number of intervals.^[1]

Properties

The sum and product of two step functions is again a step function. The product of a step function with a number is also a step function. As such, the step functions form an algebra over the real numbers.
A step function takes only a finite number of values. If the intervals $A_{i},$ $i=0,1,\dots ,n,$ in the above definition of the step function are disjoint and their union is the real line, then $f(x)=\alpha _{i}\,$ for all $x\in A_{i}.$
The definite integral of a step function is a piecewise linear function.
The Lebesgue integral of a step function $\textstyle f=\sum \limits _{i=0}^{n}\alpha _{i}\chi _{A_{i}}\,$ is $\textstyle \int \!f\,dx=\sum \limits _{i=0}^{n}\alpha _{i}\ell (A_{i}),\,$ where $\ell (A)$ is the length of the interval $A,$ and it is assumed here that all intervals $A_{i}$ have finite length. In fact, this equality (viewed as a definition) can be the first step in constructing the Lebesgue integral.^[2]

References

1 2 Bachman, Narici, Beckenstein. "Example 7.2.2". Fourier and Wavelet Analysis. Springer, New York, 2000. ISBN 0-387-98899-8.
↑ Weir, Alan J. "3". Lebesgue integration and measure. Cambridge University Press, 1973. ISBN 0-521-09751-7.

This article is issued from Wikipedia - version of the 9/2/2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.