Antiparallel (mathematics)

In geometry, anti-parallel lines can be defined with respect to either lines or angles.

Definitions

Given two lines $m_{1}\,$ and $m_{2}\,$ , lines $l_{1}\,$ and $l_{2}\,$ are anti-parallel with respect to $m_{1}\,$ and $m_{2}\,$ if $\angle 1=\angle 2\,$ in Fig.1. If $l_{1}\,$ and $l_{2}\,$ are anti-parallel with respect to $m_{1}\,$ and $m_{2}\,$ , then $m_{1}\,$ and $m_{2}\,$ are also anti-parallel with respect to $l_{1}\,$ and $l_{2}\,$ .

In any quadrilateral inscribed in a circle, any two opposite sides are anti-parallel with respect to the other two sides (Fig.2).

Two lines $l_{1}$ and $l_{2}$ are antiparallel with respect to the sides of an angle if and only if they make the same angle $\angle APC$ in the opposite senses with the bisector of that angle (Fig.3).

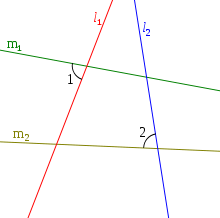Fig.1: Given two lines

m_{1}\,

and

m_{2}\,

, lines

l_{1}\,

and

l_{2}\,

are anti-parallel with respect to

m_{1}\,

and

m_{2}\,

\angle 1=\angle 2\,

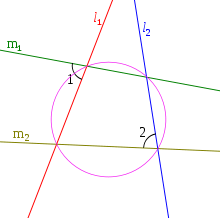Fig.2: In any quadrilateral inscribed in a circle, any two opposite sides are anti-parallel with respect to the other two sides.

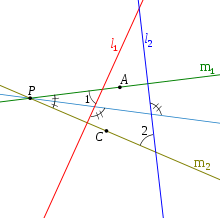Fig.3: Two lines

l_{1}\,

and

l_{2}\,

are said to be antiparallel with respect to the sides of an angle if they make the same angle

\angle APC

in the opposite senses with the bisector of that angle. Notice that our previous angles 1 and 2 are still equivalent.

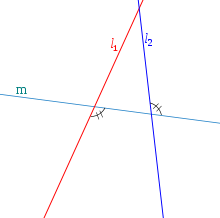Fig.4: If the lines

m_{1}\,

and

m_{2}\,

coincide,

l_{1}\,

and

l_{2}\,

are said to be anti-parallel with respect to a straight line.

Antiparallel vectors

In a Euclidean space, two directed line segments, often called vectors in applied mathematics, are antiparallel, if they are supported by parallel lines and have opposite directions.^[1] In that case, one of the associated Euclidean vectors is the product of the other by a negative number.

Relations

The line joining the feet to two altitudes of a triangle is antiparallel to the third side.(any cevians which 'see' the third side with the same angle create antiparallel lines)
The tangent to a triangle's circumcircle at a vertex is antiparallel to the opposite side.
The radius of the circumcircle at a vertex is perpendicular to all lines antiparallel to the opposite sides.

References

↑ Harris, John; Harris, John W.; Stöcker, Horst (1998). Handbook of mathematics and computational science. Birkhäuser. p. 332. ISBN 0-387-94746-9. , Chapter 6, p. 332

A.B. Ivanov, Encyclopaedia of Mathematics - ISBN 1-4020-0609-8
Weisstein, Eric W. "Antiparallel." From MathWorld--A Wolfram Web Resource.

This article is issued from Wikipedia - version of the 10/17/2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.