Barker code
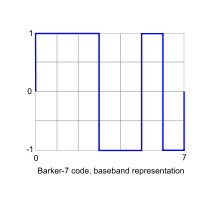
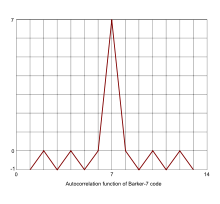
A Barker code or Barker sequence is a finite sequence of N values of +1 and −1,
with the ideal autocorrelation property, such that the off-peak (non-cyclic) autocorrelation coefficients
are as small as possible:
for all .[1]
Only nine[2] Barker sequences are known, all of length N at most 13.[3] Barker's 1953 paper asked for sequences with the stronger condition
Only four such sequences are known, shown in bold in the table below.[4] http://paper.uscip.us/jaece/JAECE.2014.1003.pdf
Known Barker codes
Here is a table of all known Barker codes, where negations and reversals of the codes have been omitted. A Barker code has a maximum autocorrelation sequence which has sidelobes no larger than 1. It is generally accepted that no other perfect binary phase codes exist.[5][6] (It has been proven that there are no further odd-length codes,[7] nor even-length codes of N < 1022.[8])
| Length | Codes | Sidelobe level ratio[9][10] | |
|---|---|---|---|
| 2 | +1 −1 | +1 +1 | −6 dB |
| 3 | +1 +1 −1 | −9.5 dB | |
| 4 | +1 +1 −1 +1 | +1 +1 +1 −1 | −12 dB |
| 5 | +1 +1 +1 −1 +1 | −14 dB | |
| 7 | +1 +1 +1 −1 −1 +1 −1 | −16.9 dB | |
| 11 | +1 +1 +1 −1 −1 −1 +1 −1 −1 +1 −1 | −20.8 dB | |
| 13 | +1 +1 +1 +1 +1 −1 −1 +1 +1 −1 +1 −1 +1 | −22.3 dB | |
Barker codes of length N equal to 11 and 13 (![]() A011758
A011758 ![]() A011759) are used in direct-sequence spread spectrum and pulse compression radar systems because of their low autocorrelation properties (The sidelobe level of amplitude of the Barker codes is 1/N that of the peak signal).[11] A Barker code resembles a discrete version of a continuous chirp, another low-autocorrelation signal used in other pulse compression radars.
A011759) are used in direct-sequence spread spectrum and pulse compression radar systems because of their low autocorrelation properties (The sidelobe level of amplitude of the Barker codes is 1/N that of the peak signal).[11] A Barker code resembles a discrete version of a continuous chirp, another low-autocorrelation signal used in other pulse compression radars.
The positive and negative amplitudes of the pulses forming the Barker codes imply the use of biphase modulation or binary phase-shift keying; that is, the change of phase in the carrier wave is 180 degrees.
Similar to the Barker codes are the complementary sequences, which cancel sidelobes exactly when summed; the even-length Barker code pairs are also complementary pairs. There is a simple constructive method to create arbitrarily long complementary sequences.
For the case of cyclic autocorrelation, other sequences have the same property of having perfect (and uniform) sidelobes, such as prime-length Legendre sequences and maximum length sequences (MLS). Arbitrarily long cyclic sequences can be constructed.
Barker modulation
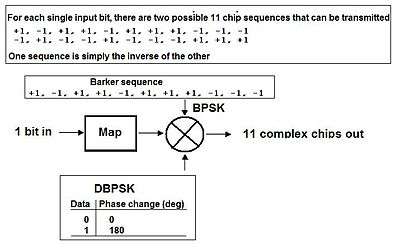
In wireless communications, sequences are usually chosen for their spectral properties and for low cross correlation with other sequences likely to interfere. In the 802.11b standard, an 11-chip Barker sequence is used for the 1 and 2 Mbit/sec rates. The value of the autocorrelation function for the Barker sequence is 0 or −1 at all offsets except zero, where it is +11. This makes for a more uniform spectrum, and better performance in the receivers.[12]
References
- ↑ Barker, R. H. (1953). "Group Synchronizing of Binary Digital Sequences". Communication Theory. London: Butterworth. pp. 273–287.
- ↑ https://oeis.org/A091704
- ↑ Borwein, Peter; Mossinghoff, Michael J. (2008). "Barker sequences and flat polynomials". In James McKee; Chris Smyth. Number Theory and Polynomials. LMS Lecture Notes. 352. Cambridge University Press. pp. 71–88. ISBN 978-0-521-71467-9.
- ↑ Using different pulse shape in Barker code also improves certain Autocorrelation properties.
- ↑ Weisstein, Eric W. "Barker Code". MathWorld.
- ↑ http://www.math.wpi.edu/MPI2008/TSC/TSC-MPI.pdf
- ↑ Turyn and Storer, "On binary sequences", Proceedings of the AMS, volume 12 (1961), pages 394–399
- ↑ Leung, K., and Schmidt, B., "The Field descent method", Design, Codes and Cryptography, volume 36, pages 171–188
- ↑ http://www.radartutorial.eu/08.transmitters/Intrapulse%20Modulation.en.html
- ↑ http://www.cacs.louisiana.edu/~library/TR/TR_pdf/TR_2006/TR_darwich_2006-4-1.pdf
- ↑ Introduction to Radar Systems, 3rd Edition, Merrill I. Skolnik, McGraw–Hill, 2001
- ↑ "802.11 & 802.11b" cp.literature.agilent.com
