Power function

For the Lego Technic motor system, see Lego Technic § Power Functions.

In mathematics, a power function is a function of the form $f(x)=x^p$ where $p$ is constant and $x$ is a variable.^[1] In general, $p$ can belong to one of several classes of numbers, such as the positive and negative integers.^[2] They are a fundamental concept in algebra and pre-calculus, leading up to the formation of polynomials.^[3] Their general form is $f(x)=cx^{p}$ , where $c$ is also a constant.

Trivial cases

Power functions with

p=1,3

and 5.

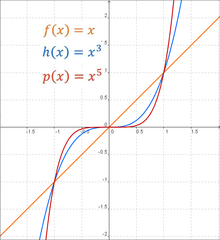
When $c=0$ , $f(x)$ is the constant function $f(x)=0$ for all real $x$ . Graphically, it is a horizontal line at $y=0$ , and can be thought of as the function that maps all inputs to 0, the number zero.

When $p=0$ , $f(x)$ is the constant function $f(x)=1$ for all real $x$ . Graphically, it is a horizontal line at $y=1$ , and can be thought of similarly to the previous case; it simply maps all inputs to 1, the number one.

When $p=1$ , $f(x)$ is the identity function $f(x)=x$ . Graphically, it is a line through the origin with slope 1, and can be thought of as the function that maps every input to itself.

Integer cases where $p\geq 2$

Power functions with

p=2,4

and 6.

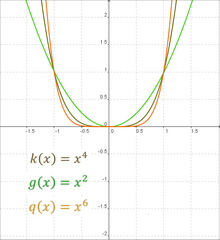
When $p$ is in $\mathbb {Z}$ and $p\geq 2$ , two primary families exist: when $p$ is even, and when $p$ is odd. In general, when $p$ is even and $x$ is large, $f(x)$ will tend towards positive infinity if $c\geq 0$ , and toward negative infinity if $c\leq 0$ . All even power function graphs of this family have the general shape of $y=x^{2}$ , flattening more in the middle as $p$ increases.^[4] Functions with this kind of symmetry are called even functions.

When $p$ is odd, $f(x)$ 's asymptotic behavior reverses from positive $x$ to negative $x$ . For $c\geq 0$ , and large and positive $x$ , $f(x)$ will tend towards positive infinity, while for large and negative $x$ , $f(x)$ will tend towards negative infinity. For $c\leq 0$ , the opposite is true in each case. All odd power function graphs of this family have the general shape of $y=x^{3}$ , flattening more in the middle as $p$ increases.^[5] Functions with this kind of symmetry are called odd functions.

Integer cases where $p<0$

When $p$ is in $\mathbb {Z}$ and $p<0$ , $f(x)$ takes the shape of a hyperbola. As with positive integers, there exist two primary families according to $p$ 's parity. Regardless of said parity, however, these families all tend toward zero for large $x$ , whether positive or negative.^[6] Where their behavior differs is in approaching $x=0$ from the right and left.

When $p$ is even, $f(x)$ is even, and thus symmetric about the $y$ -axis.^[7] Thus, when approaching $x=0$ from either the right or the left, $f(x)$ will tend towards positive infinity when $c$ is positive, and negative infinity when $c$ is negative.

When $p$ is odd, $f(x)$ is odd, and thus symmetric about the origin.^[8]

Generalizations

Polynomials, another fundamental algebraic construct, can be seen as being created from multiple power functions and their coefficient terms (i.e. $cx^{n}$ ), added together.^[9] For example, $f(x)=x^{2}+2x+1$ or $f(x)=x+x^{3}$ .

Power functions are a special case of power law relationships, which appear throughout mathematics and statistics.

References

↑ Anton, Howard; Bivens, Irl; Davis, Stephen. Calculus: Early Transcendentals (9th ed.). John Wiley & Sons. p. 28. |access-date= requires |url= (help)
↑ Anton, Howard; Bivens, Irl; Davis, Stephen. Calculus: Early Transcendentals (9th ed.). John Wiley & Sons. pp. 28–29. |access-date= requires |url= (help)
↑ Anton, Howard; Bivens, Irl; Davis, Stephen. Calculus: Early Transcendentals (9th ed.). John Wiley & Sons. p. 30. |access-date= requires |url= (help)
↑ Anton, Howard; Bivens, Irl; Davis, Stephen. Calculus: Early Transcendentals (9th ed.). John Wiley & Sons. p. 28. |access-date= requires |url= (help)
↑ Anton, Howard; Bivens, Irl; Davis, Stephen. Calculus: Early Transcendentals (9th ed.). John Wiley & Sons. p. 28. |access-date= requires |url= (help)
↑ Anton, Howard; Bivens, Irl; Davis, Stephen. Calculus: Early Transcendentals (9th ed.). John Wiley & Sons. p. 29. |access-date= requires |url= (help)
↑ Anton, Howard; Bivens, Irl; Davis, Stephen. Calculus: Early Transcendentals (9th ed.). John Wiley & Sons. p. 29. |access-date= requires |url= (help)
↑ Anton, Howard; Bivens, Irl; Davis, Stephen. Calculus: Early Transcendentals (9th ed.). John Wiley & Sons. p. 29. |access-date= requires |url= (help)
↑ Anton, Howard; Bivens, Irl; Davis, Stephen. Calculus: Early Transcendentals (9th ed.). John Wiley & Sons. p. 31. |access-date= requires |url= (help)

This article is issued from Wikipedia - version of the 8/27/2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.