Simplicial commutative ring

In algebra, a simplicial commutative ring is a commutative monoid in the category of simplicial abelian groups, or, equivalently, a simplicial object in the category of commutative rings. If A is a simplicial commutative ring, then it can be shown that 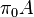\pi_0 A is a commutative ring and 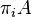\pi_i A are modules over that ring (in fact, 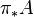\pi_* A is a graded ring over \pi_0 A.)

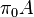A topology-counterpart of this notion is a commutative ring spectrum.

Graded ring structure

Let A be a simplicial commutative ring. Then the ring structure of A gives 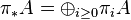\pi_* A = \oplus_{i \ge 0} \pi_i A the structure of a graded-commutative graded ring as follows.

By the Dold–Kan correspondence, 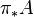\pi_* A is the homology of the chain complex corresponding to A; in particular, it is a graded abelian group. Next, to multiply two elements, writing 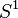S^1 for the simplicial circle, let 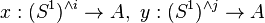x:(S^1)^{\wedge i} \to A, \, \, y:(S^1)^{\wedge j} \to A be two maps. Then the composition

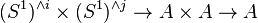(S^1)^{\wedge i} \times (S^1)^{\wedge j} \to A \times A \to A,

the second map the multiplication of A, induces 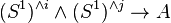(S^1)^{\wedge i} \wedge (S^1)^{\wedge j} \to A. This in turn gives an element in 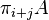\pi_{i + j} A. We have thus defined the graded multiplication 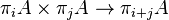\pi_i A \times \pi_j A \to \pi_{i + j} A. It is associative since the smash product is. It is graded-commutative (i.e., 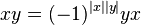xy = (-1)^{|x||y|} yx) since the involution 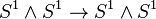S^1 \wedge S^1 \to S^1 \wedge S^1 introduces minus sign.

If M is a simplicial module over A (that is, M is a simplicial abelian group with an action of A), then the similar argument shows that 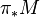\pi_* M has the structure of a graded module over 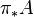\pi_* A. (cf. module spectrum.)

Spec

By definition, the category of affine derived schemes is the opposite category of the category of simplicial commutative rings; an object corresponding to A will be denoted by 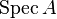\operatorname{Spec} A.

See also

References

This article is issued from Wikipedia - version of the 10/31/2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.