Neutral third

Neutral third
Inverse neutral sixth
Name
Other names -
Abbreviation n3
Size
Semitones ~3½
Interval class ~3½
Just interval 11:9,[1] 27:22,[1][2] or 16:13[3]
Cents
Equal temperament 350
24 equal temperament 350
Just intonation 347, 355, or 359
Neutral third on C  Play ,  Play 27/22 .
Neutral third

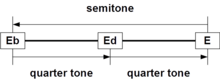
A neutral third is a musical interval wider than a minor third  play  but narrower than a major third  play , named by Jan Pieter Land in 1880;[4] the name has been taken over by Alois Hába.[5] Three distinct intervals may be termed neutral thirds:

These intervals are all within about 12 cents and are difficult for most people to distinguish by ear. Neutral thirds are roughly a quarter tone sharp from 12 equal temperament minor thirds and a quarter tone flat from 12-ET major thirds. In just intonation, as well as in tunings such as 31-ET, 41-ET, or 72-ET, which more closely approximate just intonation, the intervals are closer together.

A neutral third can be formed by stacking a neutral second together with a whole tone. Zalzal's wosta, a neutral third of 354.55 cents, may be constructed through the addition of a whole tone and a three quarter tone: 9/8 X 12/11 = 27/22.[8] Based on its positioning in the harmonic series, the undecimal neutral third implies a root one whole tone below the lower of the two notes.

A triad formed by two neutral thirds is neither major nor minor, thus the neutral thirds triad is ambiguous. While it is not found in twelve tone equal temperament it is found in others such as the quarter tone scale  Play  and 31-tet  Play .

Occurrence in human music

In infants' song

Infants experiment with singing, and a few studies of individual infants' singing found that neutral thirds regularly arise in their improvisations. In two separate case studies of the progression and development of these improvisations, neutral thirds were found to arise in infants' songs after major and minor seconds and thirds, but before intervals smaller than a semitone and also before intervals as large as a perfect fourth or larger.[9]

In modern classical Western music

The neutral third has been used by a number of modern composers, including Charles Ives, James Tenney, and Gayle Young.[10]

In traditional music

The equal-tempered neutral third may be found in the quarter tone scale and in some traditional Arab music (see also Arab tone system). Undecimal neutral thirds appear in traditional Georgian music.[11] Neutral thirds are also found in American folk music.[12]

Blue notes (a note found in country music, blues, and some rock music) on the third note of a scale can be seen as a variant of a neutral third with the tonic, as they fall in between a major third and a minor third. Similarly the blue note on the seventh note of the scale can be seen as a neutral third with the dominant. Unlike most classical music, blue notes do not have exact values.

In equal temperaments

Two steps of seven-tone equal temperament form an interval of 342.8571 cents, which is within 5 cents of 347.4079 for the undecimal (11:9) neutral third.[13] This is an equal temperament in reasonably common use, at least in the form of "near seven equal", as it is a tuning used for Thai music as well as the Ugandan Chopi tradition of music.[14]

The neutral third also has good approximations in other commonly used equal temperaments including 24-ET (7 steps, 350 cents[15]) and similarly by all multiples of 24 equal steps such as 48-ET[16] and 72-ET,[17] 31-ET (9 steps, 349.39),[18] 34-ET (10 steps, 352.941 cents[19]), 41-ET (12 steps, 351.22 cents[20]), and slightly less closely by 53-ET (15 steps, 339.62 cents[21]).

Close approximations to the tridecimal neutral third (16:13) appear in 53-ET [21] and 72-ET.[17] Both of these temperaments distinguish between the tridecimal (16:13) and undecimal (11:9) neutral thirds. All the other tuning systems mentioned above fail to distinguish between these intervals; they temper out the comma 144:143.

See also

References

  1. 1 2 Haluska, Jan (2003). The Mathematical Theory of Tone Systems, p.xxiii. ISBN 0-8247-4714-3. Undecimal neutral third and Zalzal's wosta.
  2. ↑ "Neutral third scales", Xenharmonic.Wikispaces.com.
  3. ↑ Haluska (2003), p.xxiv. Tridecimal neutral third.
  4. ↑ J. P. Land, Over de toonladders der Arabische muziek, 1880; Recherches sur l'histoire de la gamme arabe, 1884. See Hermann Ludwig F. von Helmholtz (Alexander John Ellis, trans.) (1875), p. 281, note † (addition by Ellis).
  5. ↑ Alois Hába, Neue Harmonielehre des diatonischen, chromatischen, Viertel-, Drittel-, Sechstel- und Zwölftel-Tonsystems (Liepzig: Fr. Kistner & C.F.W. Sigel, 1927), 143. [ISBN unspecified]. Cited in Skinner, Miles Leigh (2007). Toward a Quarter-tone Syntax: Analyses of Selected Works by Blackwood, Haba, Ives, and Wyschnegradsky, p.25. ProQuest. ISBN 9780542998478.
  6. ↑ Andrew Horner, Lydia Ayres (2002). Cooking with Csound: Woodwind and Brass Recipes, p.131. ISBN 0-89579-507-8. "Super-Major Second".
  7. ↑ Jan Haluska, The Mathematical Theory of Tone Systems, CRC (2004).
  8. ↑ Hermann Ludwig F. von Helmholtz (Alexander John Ellis, trans.) (1875). "Additions by the translator", On the sensations of tone as a physiological basis for the theory of music, p.281. No ISBN specified.
  9. ↑ Nettl, Bruno "Infant Musical Development and Primitive Music" Southwestern Journal of Anthropology, Vol. 12, No. 1, pp. 87-91. (Spring, 1956)
  10. ↑ Young, Gayle "The Pitch Organization of Harmonium for James Tenney", Perspectives of New Music, Vol. 26, No. 2. pp. 204-212 (Summer, 1988)
  11. ↑ Society for the Study of Caucasia (1994). Aronson, Howard I., ed. The Annual of the Society for the Study of Caucasia. The Society. p. 93. Retrieved 14 April 2011.
  12. ↑ Boswell, George W. "The Neutral Tone as a Function of Folk-Song Text", Yearbook of the International Folk Music Council, Vol. 2, 1970, pp. 127-132 (1970)
  13. ↑ "7edo", Xenharmonic.Wikispaces.com.
  14. ↑ Morton, David (1980). "The Music of Thailand", Musics of Many Cultures, p.70. May, Elizabeth, ed. ISBN 0-520-04778-8.
  15. ↑ "24edo", Xenharmonic.Wikispaces.com.
  16. ↑ "48edo", Xenharmonic.Wikispaces.com.
  17. 1 2 "72edo", Xenharmonic.Wikispaces.com.
  18. ↑ "31edo", Xenharmonic.Wikispaces.com.
  19. ↑ "34edo", Xenharmonic.Wikispaces.com.
  20. ↑ "41edo", Xenharmonic.Wikispaces.com.
  21. 1 2 "53edo", Xenharmonic.Wikispaces.com.
This article is issued from Wikipedia - version of the 10/28/2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.